Home>Mathematics>Master The Art Of Simplifying Square Roots, Division, And Multiplication!
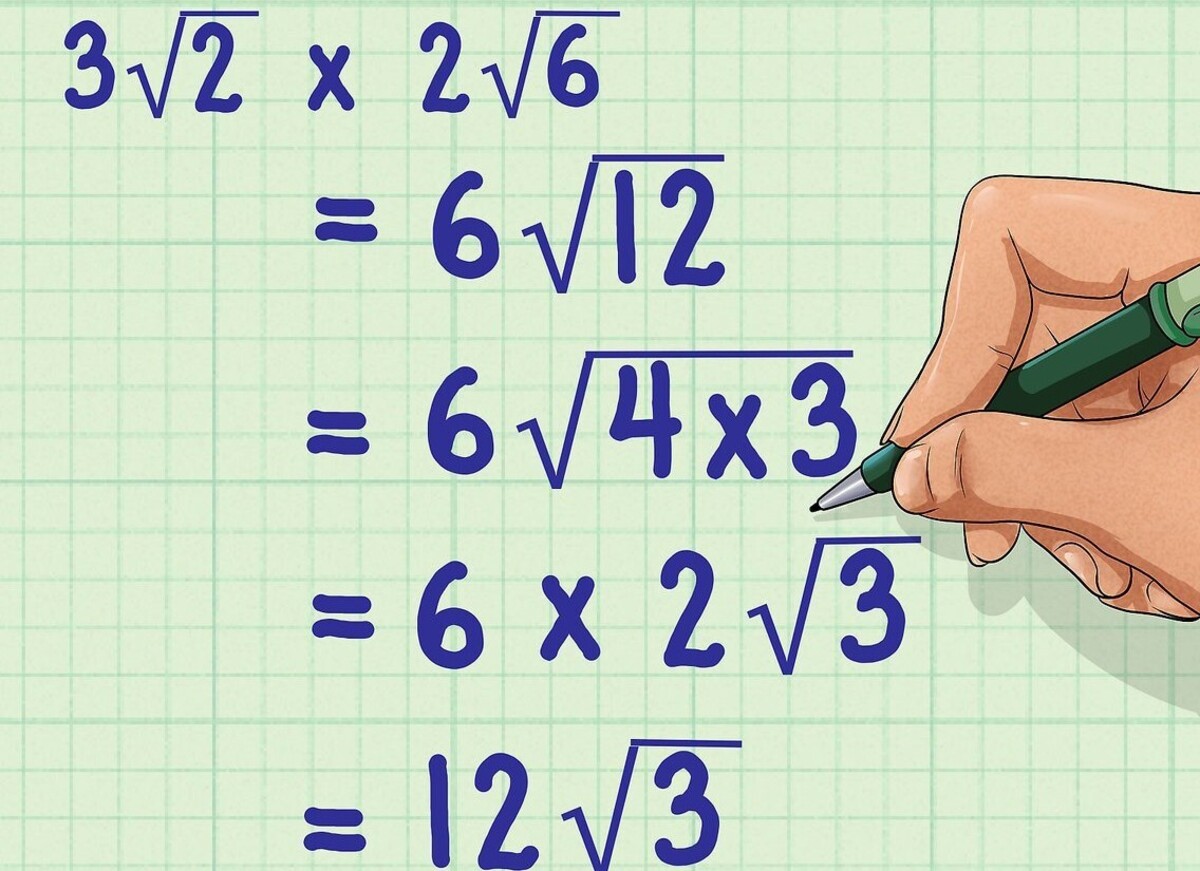
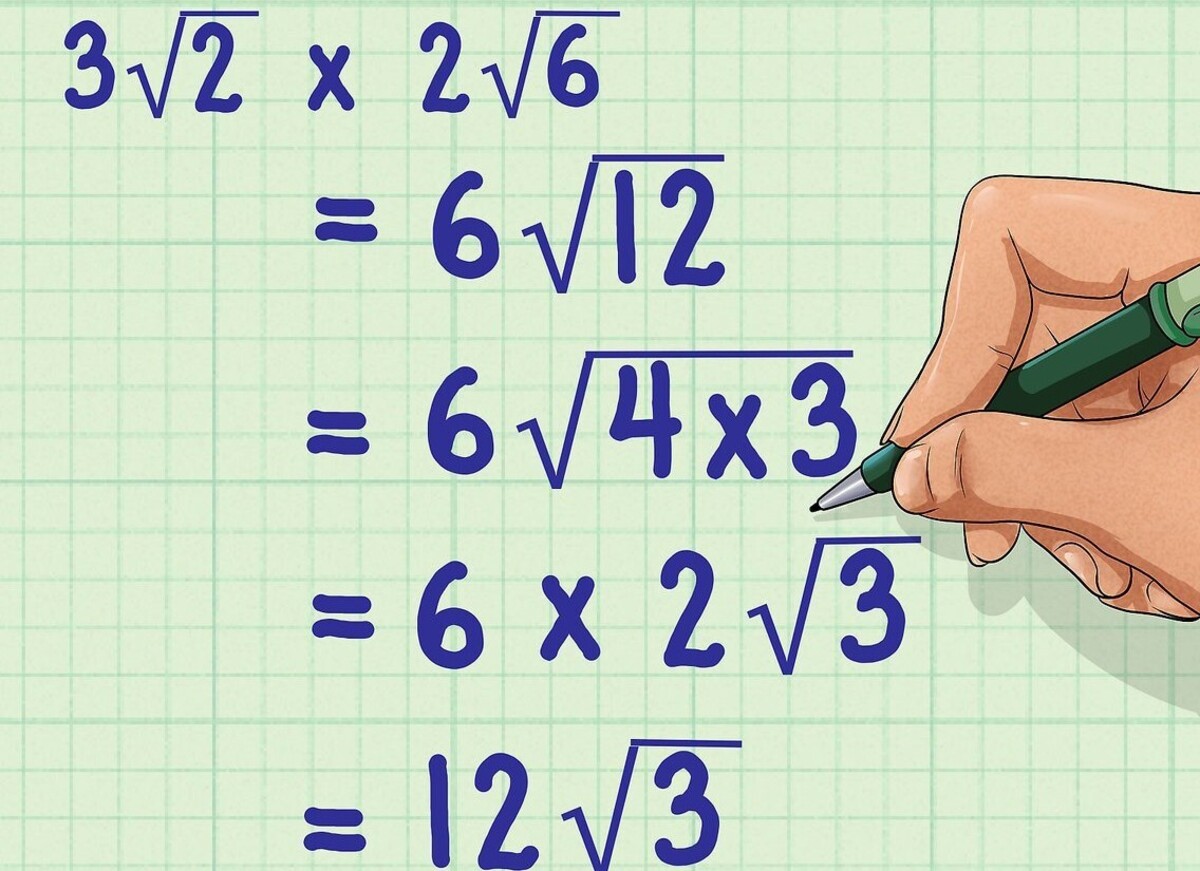
Mathematics
Master The Art Of Simplifying Square Roots, Division, And Multiplication!
Published: January 20, 2024
Learn how to simplify square roots, division, and multiplication with expert tips and techniques in mathematics. Master the art of mathematical simplification today!
(Many of the links in this article redirect to a specific reviewed product. Your purchase of these products through affiliate links helps to generate commission for Noodls.com, at no extra cost. Learn more)
Table of Contents
Simplifying Square Roots
Simplifying square roots is a fundamental concept in mathematics, and mastering this skill can significantly enhance problem-solving abilities. Square roots are essentially the opposite of squaring a number, and they are represented by the radical symbol (√). When simplifying square roots, the goal is to express the radical in its simplest form, typically by factoring the number under the radical sign into its prime factors.
Here are the essential steps to simplify square roots:
-
Identify Perfect Squares: Begin by identifying any perfect square factors within the number under the radical. Perfect squares are numbers that are the result of multiplying a number by itself, such as 4, 9, 16, and so on.
-
Factor the Number: Next, factor the number under the radical into its prime factors. This involves breaking down the number into its smallest prime factors, which are the building blocks of the original number.
-
Pair Up Factors: Once the number is factored, pair up identical factors. For each pair of identical factors, one factor can be taken out of the radical, leaving its counterpart inside.
-
Simplify: Finally, simplify the expression by taking out any factors that can be extracted from the radical. The remaining factors inside the radical represent the simplified form of the square root.
For example, let's simplify the square root of 72:
-
Identify Perfect Squares: 72 contains the perfect square factor 36 (which is 6 x 6).
-
Factor the Number: Breaking down 72 into its prime factors yields 2 x 2 x 2 x 3 x 3.
-
Pair Up Factors: Pairing up the identical factors gives us 2 x 2 and 3 x 3.
-
Simplify: Taking out the pairs of identical factors results in 6√2.
By following these steps, simplifying square roots becomes more manageable and allows for the efficient manipulation of radical expressions in various mathematical contexts. This foundational skill serves as a building block for more advanced mathematical concepts, making it a crucial aspect of mathematical proficiency.
Read more: How To Use The Square Root Symbol
Division Techniques
Division is a fundamental arithmetic operation that involves the distribution of a quantity into equal parts. When it comes to mathematical problem-solving, mastering division techniques is essential for simplifying complex expressions and solving real-world problems. In the realm of mathematics, there are several effective techniques for approaching division, each serving a unique purpose and catering to specific scenarios.
Long Division
Long division is a widely used technique for dividing large numbers or polynomials. It provides a systematic approach to dividing a dividend by a divisor, resulting in the quotient and remainder. The process involves several steps, including dividing, multiplying, subtracting, and bringing down the next digit. Long division is particularly useful when dealing with multi-digit numbers and is a fundamental skill taught in elementary mathematics education.
Synthetic Division
Synthetic division is a specialized technique used to divide polynomials by linear binomials of the form (x – k). This method offers a more concise and efficient approach compared to long division, especially for dividing polynomials by linear factors. Synthetic division is particularly valuable in algebraic contexts, allowing for the swift computation of quotients and remainders when dividing polynomials.
Fraction Division
When dividing fractions, the reciprocal of the divisor is multiplied by the dividend, transforming the division operation into a multiplication problem. This technique simplifies the process of dividing fractions and aligns with the concept of reciprocals in mathematics. Understanding fraction division is crucial for solving various real-world problems involving proportional relationships, measurements, and scaling.
Decimal Division
Decimal division involves dividing numbers with decimal representations. This technique often requires aligning the decimal points and performing division as usual. Decimal division is commonly encountered in everyday calculations, such as computing measurements, currency conversions, and solving practical mathematical problems.
Division by Powers of 10
Dividing by powers of 10 involves shifting the decimal point to the left by the number of zeros in the power of 10. This technique simplifies division when dealing with multiples of 10, 100, 1000, and so on. It is a useful strategy for quick mental calculations and is particularly practical in scientific notation and engineering applications.
Mastering these division techniques equips individuals with the necessary tools to tackle diverse mathematical challenges with confidence and precision. Whether working with whole numbers, fractions, polynomials, or decimals, a strong command of division techniques is indispensable for navigating the complexities of mathematics and its practical applications.
Multiplication Strategies
Multiplication lies at the core of arithmetic and serves as a fundamental building block for a wide array of mathematical concepts. By mastering effective multiplication strategies, individuals can streamline calculations, solve complex problems, and gain a deeper understanding of mathematical relationships. Here, we explore various multiplication strategies that cater to diverse scenarios and mathematical contexts.
Partial Products Method
The partial products method offers a structured approach to multiplying multi-digit numbers. It involves breaking down the multiplication process into smaller, more manageable steps. By decomposing each factor into its place value components and systematically multiplying them, this method facilitates a clearer understanding of the multiplication process. The partial products method is particularly beneficial for students learning multi-digit multiplication, providing a visual and conceptual framework for mastering this essential skill.
Lattice Multiplication
Lattice multiplication provides a visual and systematic approach to multiplying multi-digit numbers. This method involves creating a grid-like structure, known as a lattice, to organize the multiplication process. By filling in the lattice with the products of the individual digits and summing the diagonal entries, this technique offers a structured and intuitive way to perform multi-digit multiplication. Lattice multiplication is especially useful for visual learners and can enhance understanding and retention of multiplication concepts.
Multiplying by Powers of 10
When multiplying numbers by powers of 10, the placement of the decimal point shifts to the right according to the number of zeros in the power of 10. This strategy simplifies the multiplication process and aligns with the concept of place value. Multiplying by powers of 10 is integral to understanding decimal multiplication and plays a crucial role in scientific notation, where numbers are expressed as the product of a decimal and a power of 10.
Area Model Multiplication
The area model multiplication approach leverages geometric representations to illustrate the multiplication of two numbers. By partitioning the numbers into smaller units and calculating the areas of the resulting rectangles, this method provides a visual and conceptual framework for understanding multiplication. The area model is particularly valuable for demonstrating the distributive property and deepening students' comprehension of the relationship between multiplication and area.
Multiplying Fractions
When multiplying fractions, the numerators and denominators are multiplied separately, resulting in the product fraction. Understanding fraction multiplication is essential for various real-world applications, such as scaling recipes, calculating proportions, and solving problems involving fractional quantities. By mastering fraction multiplication, individuals gain proficiency in manipulating fractional values and solving a diverse range of mathematical problems.
By incorporating these multiplication strategies into mathematical practice, individuals can enhance their computational efficiency, deepen their conceptual understanding, and tackle diverse mathematical challenges with confidence and precision. Whether working with whole numbers, decimals, fractions, or geometric representations, a versatile command of multiplication strategies is indispensable for navigating the complexities of mathematics and its practical applications.