Simplify 24:18 To Its Simplest Form!

(Many of the links in this article redirect to a specific reviewed product. Your purchase of these products through affiliate links helps to generate commission for Noodls.com, at no extra cost. Learn more)

Introduction

Fractions are a fundamental concept in mathematics, often used to represent parts of a whole. Understanding how to simplify fractions to their simplest form is a valuable skill that can be applied in various real-life scenarios and mathematical problems. In this article, we will explore the process of simplifying the fraction 24:18 to its simplest form. By delving into the underlying principles of fractions and employing mathematical techniques, we will unravel the steps involved in simplifying this fraction.

Simplifying fractions involves reducing them to their lowest terms, where the numerator and denominator have no common factors other than 1. This process not only aids in making calculations more manageable but also provides a clearer representation of the relationship between the parts and the whole. As we embark on this journey to simplify 24:18, we will unravel the significance of fractions in everyday life and gain a deeper appreciation for their role in mathematical problem-solving.

Join me as we delve into the world of fractions and embark on a captivating exploration of simplifying 24:18 to its simplest form. Let's unlock the secrets of fractions and discover the beauty of mathematical simplification!

Understanding Fractions

Fractions are a fundamental concept in mathematics, representing a part of a whole or a ratio between two numbers. They are a crucial tool for expressing quantities that are not whole numbers, providing a way to articulate portions, divisions, and comparisons. In essence, fractions offer a means to dissect and comprehend the relationships between different quantities, making them indispensable in various real-world scenarios.

At their core, fractions consist of two essential components: the numerator and the denominator. The numerator denotes the number of parts being considered, while the denominator represents the total number of equal parts that make up the whole. This relationship is akin to dividing a whole into equal segments, with the numerator specifying the number of these segments under consideration, and the denominator indicating the total number of segments comprising the whole.

Fractions come in various forms, including proper fractions, improper fractions, and mixed numbers. Proper fractions have numerators smaller than their denominators and represent values less than one. On the other hand, improper fractions have numerators greater than or equal to their denominators, signifying values equal to or greater than one. Mixed numbers, a combination of whole numbers and fractions, offer a more comprehensive representation of quantities, particularly in practical contexts.

Understanding fractions extends beyond mere numerical representation; it involves grasping the underlying concept of division and proportion. Fractions enable us to articulate portions of a whole in a precise and concise manner, making them invaluable in diverse fields such as cooking, construction, and finance. For instance, in a recipe calling for 3/4 cup of flour, the fraction 3/4 delineates a specific portion of the whole quantity, facilitating accurate measurement and ingredient proportioning.

Moreover, fractions serve as the building blocks for more advanced mathematical operations, laying the groundwork for concepts such as decimals, percentages, and ratios. They provide a seamless transition between whole numbers and more complex numerical representations, fostering a deeper understanding of mathematical relationships and facilitating problem-solving in various disciplines.

In essence, comprehending fractions is pivotal in navigating the intricacies of mathematics and real-world applications. By mastering the principles of fractions, individuals can effectively communicate and manipulate quantities, laying a solid foundation for advanced mathematical concepts and practical endeavors. As we delve into the process of simplifying the fraction 24:18, our understanding of fractions will serve as a cornerstone for unraveling the beauty and utility of mathematical simplification.

Finding the Greatest Common Divisor

The process of simplifying fractions hinges on the concept of the greatest common divisor (GCD), also known as the greatest common factor. The GCD of two numbers is the largest positive integer that divides both numbers without leaving a remainder. In the context of simplifying fractions, finding the GCD of the numerator and denominator is a crucial step in reducing the fraction to its simplest form.

To calculate the GCD of two numbers, various methods can be employed, with the most common approach being the prime factorization method. This method involves breaking down each number into its prime factors and identifying the common prime factors shared by both numbers. By determining the highest power of each common prime factor present in both numbers, we can ascertain the GCD.

Consider the fraction 24:18. To simplify this fraction, we must first find the GCD of the numerator (24) and the denominator (18).

Beginning with the prime factorization of 24, we express it as 2^3 * 3^1. For the number 18, the prime factorization yields 2^1 * 3^2.

Identifying the common prime factors shared by 24 and 18, we observe that both numbers contain the prime factor 2 and the prime factor 3. To determine the GCD, we take the lowest power of each common prime factor present in both numbers. In this case, the lowest power of 2 is 1, and the lowest power of 3 is also 1.

By multiplying these lowest powers together, we obtain the GCD of 24 and 18, which is 2^1 * 3^1 = 6. Therefore, the greatest common divisor of 24 and 18 is 6.

Understanding the significance of the GCD in simplifying fractions is pivotal, as it provides a systematic and reliable method for reducing fractions to their simplest form. By leveraging the GCD, we can divide both the numerator and denominator of a fraction by their common factor, resulting in a simplified fraction that accurately represents the original ratio.

As we delve deeper into the process of simplifying the fraction 24:18, our grasp of the GCD will serve as a cornerstone for unraveling the beauty and utility of mathematical simplification.

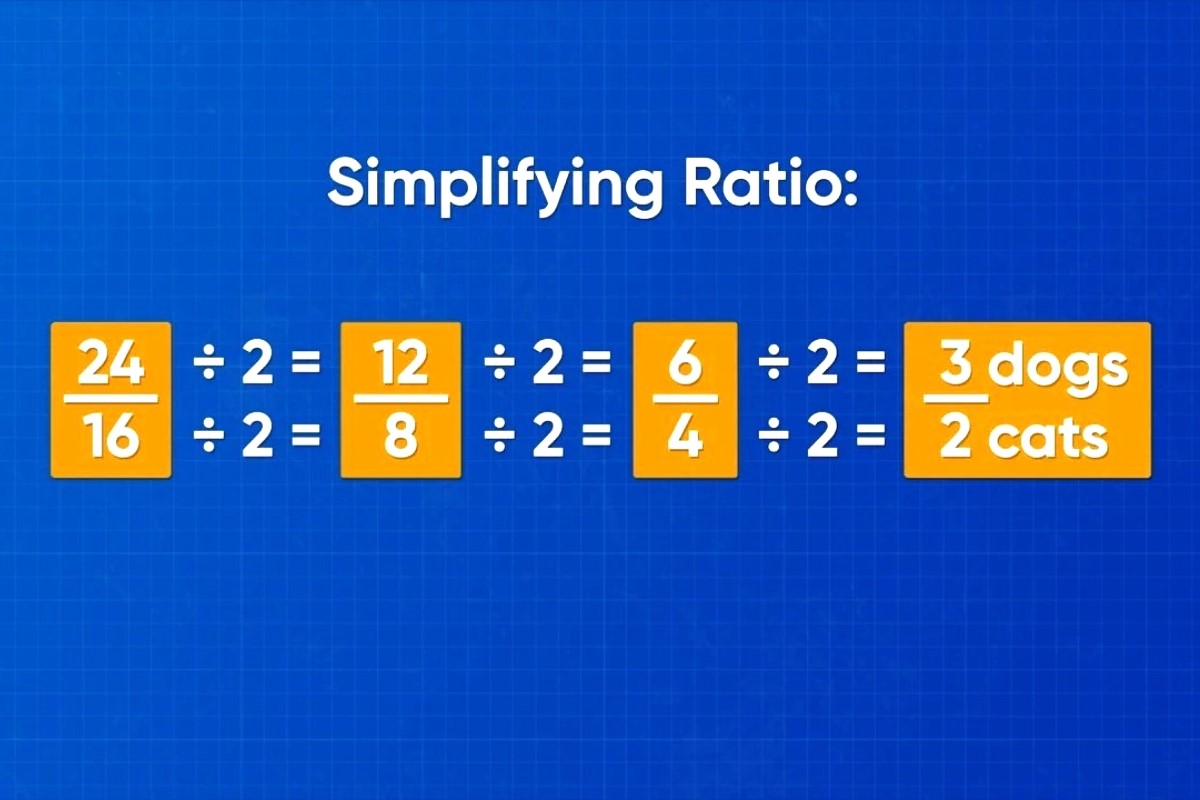
Simplifying 24:18

Now that we have explored the fundamentals of fractions and the significance of the greatest common divisor (GCD), we are poised to delve into the process of simplifying the fraction 24:18 to its simplest form. This endeavor will unveil the practical application of the GCD in reducing a fraction to its most concise representation, showcasing the elegance of mathematical simplification.

To simplify the fraction 24:18, we first ascertain the GCD of the numerator (24) and the denominator (18) using the prime factorization method. As previously established, the prime factorization of 24 yields 2^3 * 3^1, while the prime factorization of 18 results in 2^1 * 3^2. Identifying the common prime factors shared by both numbers, we recognize the presence of the prime factor 2 and the prime factor 3.

To find the GCD, we take the lowest power of each common prime factor present in both numbers. In this case, the lowest power of 2 is 1, and the lowest power of 3 is also 1. By multiplying these lowest powers together, we obtain the GCD of 24 and 18, which is 2^1 * 3^1 = 6. Therefore, the greatest common divisor of 24 and 18 is 6.

Having determined the GCD, we proceed to simplify the fraction 24:18. By dividing both the numerator and denominator by their common factor, which is the GCD of 6, we effectively reduce the fraction to its simplest form. The process unfolds as follows:

24 ÷ 6 = 4
18 ÷ 6 = 3

As a result, the fraction 24:18 simplifies to 4:3, representing the original ratio in its most reduced and concise state. This simplified form encapsulates the essence of the original fraction, conveying the relationship between the numerator and denominator with utmost clarity and precision.

The journey to simplify 24:18 has not only showcased the practical application of the GCD in mathematical simplification but has also underscored the transformative power of reducing fractions to their simplest form. By harnessing the principles of fractions and leveraging the GCD, we have unraveled the beauty and utility of mathematical simplification, paving the way for a deeper appreciation of the elegance inherent in mathematical processes.

As we conclude this exploration, the journey through the intricacies of simplifying 24:18 has shed light on the profound impact of mathematical concepts in shaping our understanding of the world around us. Let us carry forth this newfound insight, embracing the beauty of fractions and the art of simplification as we continue our mathematical odyssey.

Conclusion

In the realm of mathematics, fractions stand as pillars of precision, offering a nuanced representation of relationships and proportions. Throughout our exploration of simplifying the fraction 24:18, we have delved into the intricate tapestry of fractions, unraveling their significance in both theoretical and practical contexts. By understanding the underlying principles of fractions and harnessing the power of the greatest common divisor (GCD), we have embarked on a transformative journey that has illuminated the elegance and utility of mathematical simplification.

The process of simplifying 24:18 to its simplest form has not only showcased the practical application of the GCD but has also underscored the transformative power of reducing fractions to their most concise representation. As we navigated through the prime factorization method and harnessed the GCD to unveil the simplest form of the fraction 24:18, we witnessed the profound impact of mathematical concepts in shaping our understanding of numerical relationships and proportions.

By leveraging the GCD and systematically reducing the fraction 24:18 to its simplest form, 4:3, we have honed our ability to articulate ratios with utmost clarity and precision. This simplified form encapsulates the essence of the original fraction, distilling its essence into a compact and comprehensible representation. Through this process, we have not only deepened our grasp of fractions but have also honed our problem-solving skills, laying a solid foundation for tackling more complex mathematical challenges.

As we conclude this exploration, let us carry forth this newfound insight, embracing the beauty of fractions and the art of simplification as we continue our mathematical odyssey. The journey through the intricacies of simplifying 24:18 has shed light on the profound impact of mathematical concepts in shaping our understanding of the world around us. Let us celebrate the elegance and utility of mathematical simplification, recognizing its pervasive influence in diverse disciplines and its role in fostering a deeper appreciation for the precision and beauty inherent in mathematics.

In essence, the process of simplifying fractions transcends mere numerical manipulation; it embodies the art of distilling complex relationships into their purest form, unveiling the inherent harmony and symmetry within mathematical constructs. As we carry forward the wisdom gleaned from our exploration, let us continue to embrace the elegance of fractions and the transformative power of mathematical simplification, enriching our journey through the boundless realm of mathematics.