How To Calculate The Area Of A Circle Given The Radius

(Many of the links in this article redirect to a specific reviewed product. Your purchase of these products through affiliate links helps to generate commission for Noodls.com, at no extra cost. Learn more)

Introduction

Calculating the area of a circle is a fundamental concept in mathematics and geometry. It's a skill that not only helps us understand the properties of circles but also has practical applications in various fields, from engineering and architecture to physics and astronomy. The area of a circle is the measure of the space enclosed by its circumference, and it's a crucial calculation in many real-world scenarios.

Understanding how to calculate the area of a circle given the radius is a valuable skill that empowers individuals to solve problems involving circular shapes. Whether you're a student learning about geometry or a professional needing to make precise measurements, mastering this calculation is essential.

In this article, we will delve into the formula for the area of a circle and provide a step-by-step guide to help you effortlessly calculate it. We'll also work through some example problems to illustrate the application of the formula in different contexts. By the end of this article, you'll have a solid grasp of how to calculate the area of a circle and be equipped to tackle related challenges with confidence.

The beauty of mathematics lies in its ability to describe and quantify the world around us, and the area of a circle is a prime example of this. It's a concept that transcends theoretical knowledge and finds practical use in countless situations. So, let's embark on this journey of exploration and discovery, as we unravel the mysteries of the circle and its area.

Understanding the Formula for the Area of a Circle

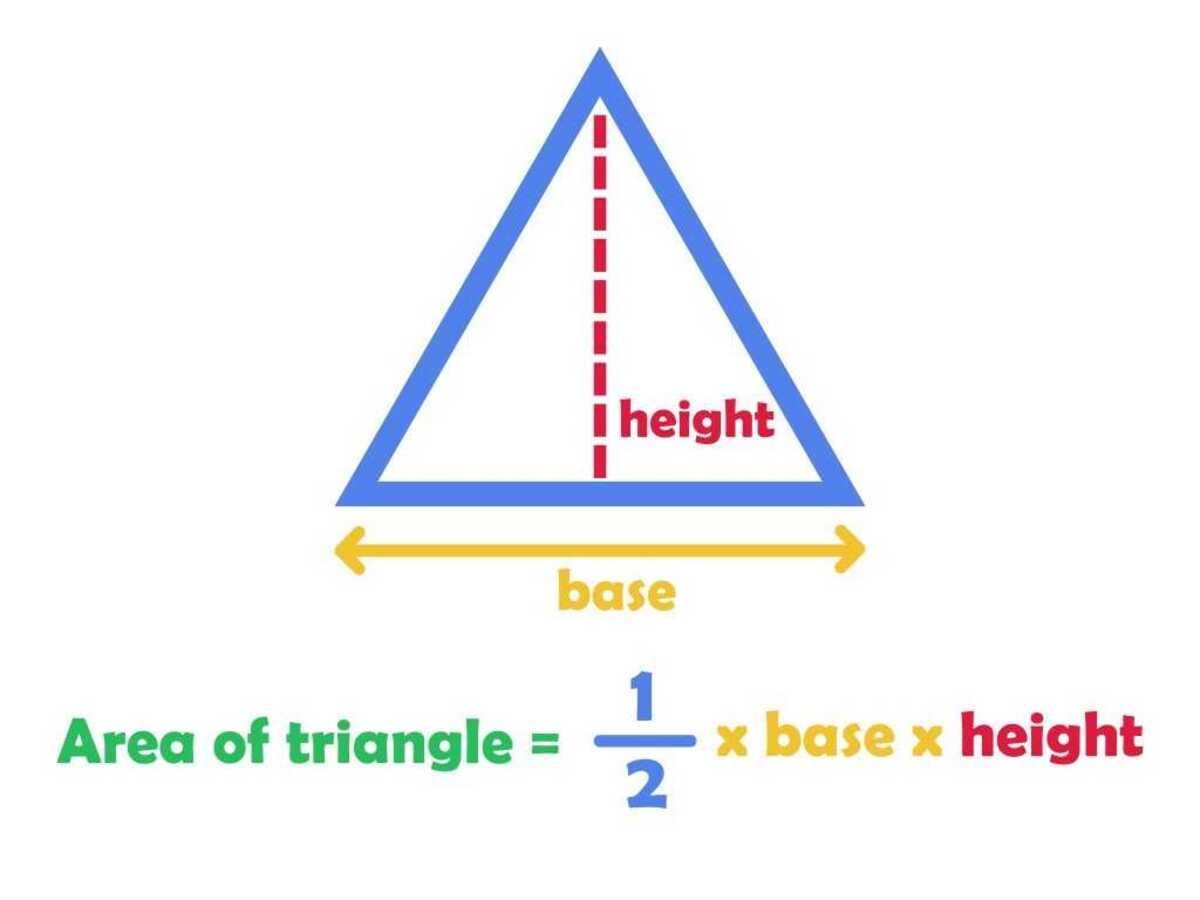
The formula for calculating the area of a circle is a fundamental concept in geometry. It provides a precise method for determining the amount of space enclosed by a circle, and it's based on the circle's radius. The radius of a circle is the distance from the center to any point on the circumference, and it plays a crucial role in the area calculation.

The formula for the area of a circle is expressed as A = πr^2, where A represents the area and r denotes the radius of the circle. The symbol π, pronounced as "pi," is a mathematical constant approximately equal to 3.14159. It's a key component in the formula and is used to account for the curvature of the circle.

Understanding the derivation of the area formula sheds light on the geometric principles behind it. The formula is derived from the concept of squaring the radius and multiplying it by π. This process effectively captures the extent of the circle's coverage in a two-dimensional space.

The significance of the formula becomes evident when considering the relationship between the radius and the area. As the radius of a circle increases, the area also increases proportionally, following a quadratic relationship. This insight underscores the direct influence of the radius on the extent of the circle's coverage, making the area formula a powerful tool for geometric analysis.

Moreover, the formula's reliance on π reflects the inherent connection between circles and the concept of pi. The value of π is a universal constant that appears in various mathematical and scientific contexts, and its presence in the area formula underscores the pervasive nature of circles in the natural world.

By comprehending the formula for the area of a circle, individuals gain a deeper appreciation for the elegance and precision of mathematical concepts. It enables them to grasp the intricate relationship between the circle's radius and its area, fostering a holistic understanding of geometric principles.

In the next section, we will delve into a step-by-step guide to calculating the area of a circle, providing a practical framework for applying the formula in real-world scenarios. This exploration will illuminate the practical significance of the area formula and equip readers with the knowledge to confidently tackle area calculations in diverse contexts.

Step-by-Step Guide to Calculating the Area of a Circle

To calculate the area of a circle, we follow a straightforward process that leverages the area formula A = πr^2. This formula encapsulates the essential relationship between the circle's radius and its area, providing a reliable method for determining the extent of space enclosed by the circle's circumference.

1. Identify the Radius: The first step is to ascertain the radius of the circle. The radius is the distance from the center of the circle to any point on its circumference. It serves as a pivotal parameter in the area calculation, influencing the magnitude of the circle's coverage.

2. Square the Radius: Once the radius is known, the next step is to square it. Squaring the radius involves multiplying the radius by itself, resulting in the radius raised to the power of 2. This process is represented as r^2 and captures the spatial extent of the circle in a two-dimensional plane.

3. Multiply by π: After obtaining the squared value of the radius, the next step is to multiply it by the mathematical constant π. This step integrates the curvature of the circle into the area calculation, accounting for the circular shape's coverage in a precise manner.

4. Apply the Area Formula: By multiplying the squared radius by π, we arrive at the area of the circle. The area formula A = πr^2 encapsulates this process, providing a direct and efficient method for calculating the space enclosed by the circle's circumference.

By following these steps, individuals can seamlessly calculate the area of a circle, leveraging the fundamental principles of geometry and the elegant simplicity of the area formula. This step-by-step guide demystifies the process of area calculation, empowering individuals to confidently tackle circular geometry problems and real-world applications.

The practical significance of this guide extends to various fields, including architecture, engineering, and physics, where precise measurements and geometric analyses are paramount. Mastering the art of calculating the area of a circle equips individuals with a valuable skill set that transcends theoretical knowledge and finds practical utility in diverse scenarios.

In the subsequent section, we will explore example problems that showcase the application of the area formula in different contexts, further illustrating the versatility and relevance of this fundamental geometric concept.

Example Problems

To reinforce our understanding of calculating the area of a circle, let's work through some example problems that showcase the practical application of the area formula in diverse scenarios. These examples will illustrate how the fundamental principles of geometry and the area formula can be employed to solve real-world problems involving circular shapes.

Example 1: Garden Design

Imagine a circular garden with a radius of 10 meters. To determine the area of the garden, we can apply the area formula A = πr^2. By substituting the given radius into the formula, we obtain:

A = π * (10)^2
A ≈ 3.14159 * 100
A ≈ 314.159 square meters

Hence, the area of the garden is approximately 314.159 square meters. This calculation provides valuable insight for landscaping and gardening purposes, enabling precise measurements of the garden's coverage and the materials required for its maintenance.

Example 2: Circular Pond

Consider a circular pond with a diameter of 6 meters. To calculate the area of the pond, we first need to determine the radius, which is half the diameter. Therefore, the radius (r) is 3 meters. Applying the area formula, we have:

A = π * (3)^2
A ≈ 3.14159 * 9
A ≈ 28.274 square meters

Thus, the area of the circular pond is approximately 28.274 square meters. This calculation is essential for pond maintenance and water volume estimation, providing valuable information for aquatic ecosystems and environmental management.

Example 3: Circular Patio

Suppose a circular patio has a circumference of 15 meters. To find the area of the patio, we can use the relationship between the circumference (C) and the radius (r), given by C = 2πr. By rearranging the formula to solve for the radius, we obtain r = C / (2π). Substituting the given circumference into the equation, we get:

r = 15 / (2 * 3.14159)
r ≈ 2.388 meters

Next, we can calculate the area using the obtained radius:

A = π * (2.388)^2
A ≈ 3.14159 * 5.7
A ≈ 17.935 square meters

Hence, the area of the circular patio is approximately 17.935 square meters. This example demonstrates the practical application of geometric relationships in determining the area of circular structures, facilitating architectural and landscaping endeavors.

By working through these example problems, we gain practical insight into the application of the area formula in diverse contexts, ranging from landscaping and environmental management to architectural design and urban planning. These examples highlight the versatility and relevance of the area formula, showcasing its pivotal role in solving real-world problems involving circular geometry.

Conclusion

In conclusion, the calculation of the area of a circle is a foundational skill that holds significant practical relevance across various disciplines. By understanding the formula A = πr^2 and following a step-by-step approach, individuals can confidently determine the extent of space enclosed by a circle's circumference. The elegance and precision of the area formula, coupled with its practical application in real-world scenarios, underscore its pivotal role in geometric analysis and problem-solving.

Mastering the art of calculating the area of a circle empowers individuals to make informed decisions in fields such as architecture, engineering, landscaping, and environmental management. Whether it's designing circular structures, estimating land coverage, or managing natural resources, the ability to calculate the area of a circle is indispensable.

Furthermore, the area formula encapsulates the inherent relationship between the circle's radius and its coverage, providing a tangible representation of geometric principles. This relationship extends beyond mathematical abstraction and finds expression in the physical world, where circular shapes abound in natural and man-made environments.

By working through example problems, we have witnessed the versatility and practical significance of the area formula. From determining garden areas to estimating water volumes in ponds and calculating the space for circular patios, the area formula serves as a reliable tool for precise measurements and geometric analyses.

In essence, the journey of exploring the area of a circle has not only enriched our understanding of geometric concepts but has also illuminated the seamless integration of mathematics into our daily lives. The ability to calculate the area of a circle is a testament to the universal applicability of mathematical principles and their profound impact on problem-solving and decision-making.

As we conclude this exploration, it's evident that the area of a circle transcends its mathematical definition, embodying a practical and aesthetic significance that resonates in diverse domains. Embracing the elegance and utility of the area formula equips individuals with a valuable skill set that extends beyond theoretical knowledge, enriching their ability to engage with the world through the lens of mathematics and geometry.