How To Find The Altitude Of A Triangle

(Many of the links in this article redirect to a specific reviewed product. Your purchase of these products through affiliate links helps to generate commission for Noodls.com, at no extra cost. Learn more)

Introduction

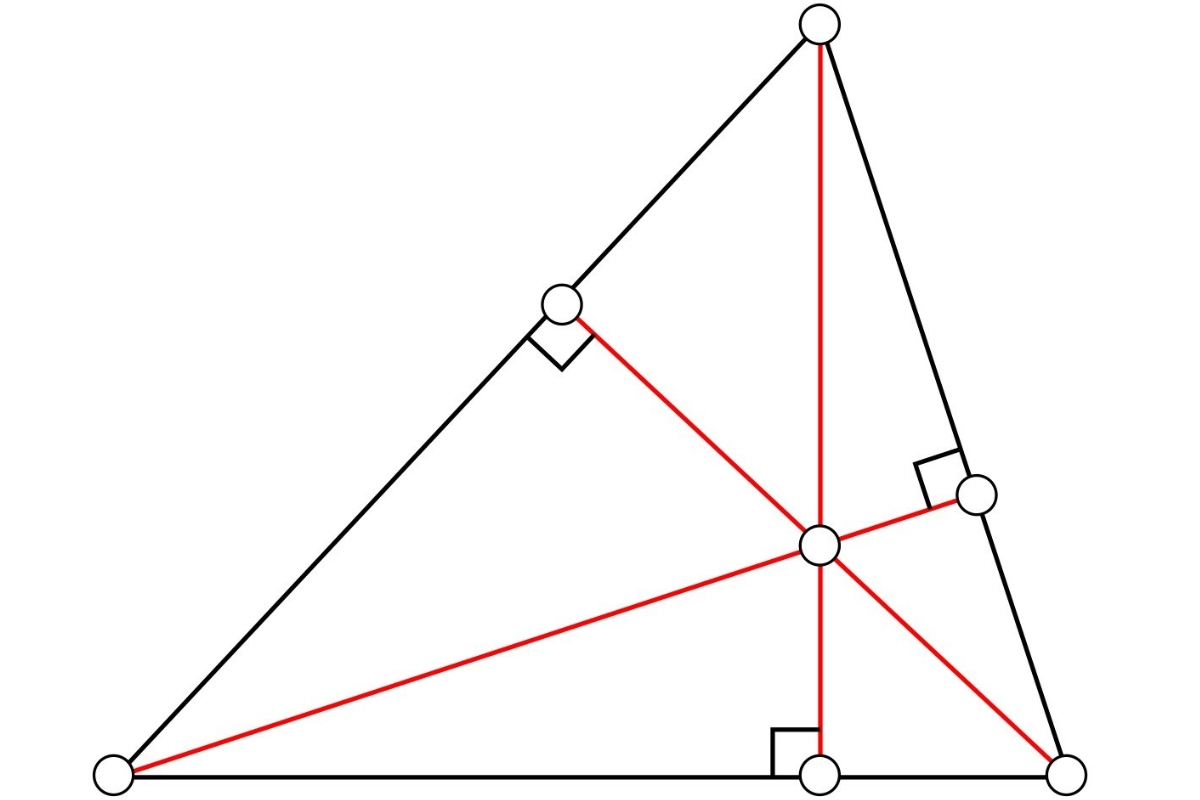
Understanding the concept of altitude in a triangle is fundamental to geometry and has practical applications in various fields such as architecture, engineering, and navigation. In geometry, the altitude of a triangle refers to the perpendicular line segment drawn from one vertex of the triangle to the opposite side, forming a right angle. This concept plays a crucial role in determining the height of objects, calculating distances, and solving real-world problems.

The altitude of a triangle is a key element in understanding the relationships between the sides and angles of the triangle. By exploring the methods for finding the altitude, we can gain insights into the principles of trigonometry and the calculation of the area of a triangle. These methods provide valuable tools for solving geometric problems and understanding the spatial relationships between different elements.

In this article, we will delve into the concept of altitude in a triangle, exploring the various methods for finding the altitude and their practical applications. By understanding the significance of the altitude in a triangle, we can appreciate its role in geometry and its relevance in real-world scenarios. Let's embark on a journey to unravel the mysteries of altitude in triangles and discover the fascinating applications of this fundamental geometric concept.

Understanding the Altitude of a Triangle

The altitude of a triangle is a fundamental concept in geometry that holds significant importance in understanding the spatial relationships within a triangle. In a triangle, the altitude refers to the perpendicular line segment drawn from one vertex to the opposite side, forming a right angle. This perpendicular line segment provides a crucial link between the vertices and the sides of the triangle, creating a framework for analyzing the triangle's properties and solving geometric problems.

When the altitude is drawn from a vertex to the opposite side, it divides the triangle into two smaller triangles. These smaller triangles share the altitude as a common side, and their areas are directly proportional to the lengths of the sides they share. This relationship forms the basis for various methods of finding the altitude of a triangle, including using trigonometric functions and the area of the triangle.

Understanding the altitude of a triangle enables us to explore the concept of height within the context of geometric shapes. Just as the altitude of a triangle represents its height, this concept extends to other geometric figures, providing a framework for understanding spatial dimensions and relationships. By visualizing the altitude as a vertical measurement within the triangle, we can grasp the concept of height in a geometric context, laying the foundation for solving problems related to distances, dimensions, and spatial configurations.

Moreover, the altitude of a triangle plays a crucial role in determining the perpendicular distance from a vertex to the opposite side, providing insights into the relative positions of different elements within the triangle. This understanding forms the basis for calculating the area of a triangle, as the altitude serves as a key component in the formula for determining the triangle's area. By comprehending the significance of the altitude, we can unlock the geometric properties of triangles and apply this knowledge to practical scenarios in various fields.

In essence, understanding the altitude of a triangle is essential for grasping the spatial relationships and dimensions within geometric shapes. It forms the basis for exploring the height of triangles and serves as a fundamental concept in geometry, paving the way for solving problems related to distances, areas, and spatial configurations. By delving into the intricacies of the altitude of a triangle, we can gain valuable insights into the geometric properties of triangles and their practical applications in diverse fields.

Methods for Finding the Altitude of a Triangle

Using Trigonometry to Find the Altitude

Trigonometry provides a powerful method for finding the altitude of a triangle by leveraging the relationships between the angles and sides of the triangle. When the length of a side and the measures of the angles are known, trigonometric functions such as sine, cosine, and tangent can be employed to calculate the altitude. Specifically, the sine function can be utilized to determine the altitude from a vertex to the opposite side, providing a precise measurement of the perpendicular distance.

By applying the sine function to the given angle, the length of the side opposite the angle, and the altitude, we can establish a relationship that enables us to solve for the altitude. This method is particularly useful when working with right-angled triangles, as the sine function directly relates the angle and the ratio of the opposite side to the hypotenuse. Through trigonometric calculations, the altitude of the triangle can be accurately determined, offering a systematic approach to solving geometric problems involving triangles.

Using the Area of the Triangle to Find the Altitude

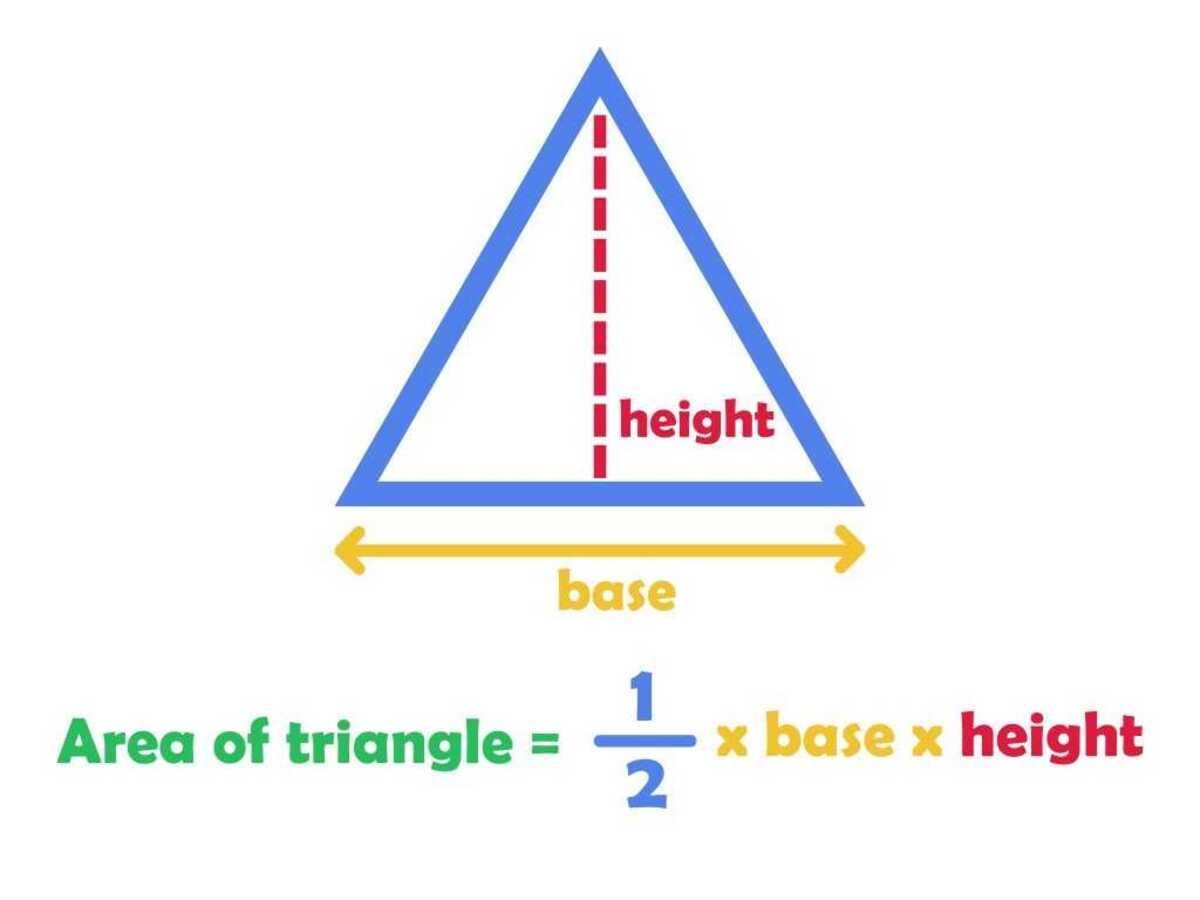
Another method for finding the altitude of a triangle involves utilizing the formula for the area of a triangle. By rearranging the formula, which incorporates the base and the height (altitude) of the triangle, we can solve for the altitude. This approach is particularly effective when the base and the corresponding height are known, allowing for the direct calculation of the altitude using the area formula.

The area of a triangle can be calculated using various methods, such as the formula A = 0.5 * base * height, where the base represents the length of the side to which the altitude is drawn. By rearranging this formula to solve for the height (altitude), we can obtain a precise measurement of the altitude. This method provides a straightforward and practical approach to finding the altitude of a triangle, leveraging the fundamental relationship between the area, base, and height of the triangle.

By employing these methods for finding the altitude of a triangle, we can harness the power of trigonometry and the area formula to determine the perpendicular distance from a vertex to the opposite side. These systematic approaches offer valuable tools for solving geometric problems and gaining insights into the spatial relationships within triangles. Through the application of trigonometric functions and the area formula, we can unravel the mysteries of altitude in triangles and apply this knowledge to practical scenarios in diverse fields.

Using Trigonometry to Find the Altitude

Trigonometry serves as a powerful tool for determining the altitude of a triangle by leveraging the relationships between the angles and sides of the triangle. When equipped with the length of a side and the measures of the angles, trigonometric functions such as sine, cosine, and tangent come into play to calculate the altitude. Specifically, the sine function plays a pivotal role in establishing the altitude from a vertex to the opposite side, providing an accurate measurement of the perpendicular distance within the triangle.

In the context of right-angled triangles, the sine function offers a direct relationship between the angle and the ratio of the opposite side to the hypotenuse. This fundamental connection forms the basis for utilizing trigonometric calculations to determine the altitude of the triangle. By applying the sine function to the given angle, the length of the side opposite the angle, and the altitude, a precise relationship is established, enabling the calculation of the altitude.

The systematic application of trigonometric functions to find the altitude of a triangle offers a structured approach to solving geometric problems. It provides a means to accurately determine the perpendicular distance from a vertex to the opposite side, thereby unlocking the spatial dimensions and relationships within the triangle. This method not only enhances the understanding of the geometric properties of triangles but also equips individuals with the tools to solve real-world problems that involve spatial configurations and distances.

By delving into the intricacies of trigonometry and its application in finding the altitude of a triangle, individuals can gain valuable insights into the geometric principles that govern spatial relationships. This method offers a systematic and precise approach to determining the altitude, laying the foundation for solving geometric problems with confidence and accuracy. Through the utilization of trigonometric functions, the altitude of a triangle becomes a tangible and calculable element, providing a gateway to understanding the spatial dimensions and configurations within geometric shapes.

In essence, the application of trigonometry to find the altitude of a triangle offers a methodical and structured approach to solving geometric problems. By leveraging the relationships between angles and sides, individuals can harness the power of trigonometric functions to determine the altitude, unlocking the spatial dimensions and relationships within triangles. This method not only enriches the understanding of geometry but also equips individuals with valuable tools for solving practical problems in diverse fields.

Using the Area of the Triangle to Find the Altitude

Utilizing the area of a triangle to find the altitude provides a practical and insightful method for determining the perpendicular distance from a vertex to the opposite side. This approach leverages the fundamental relationship between the area, base, and height of the triangle, offering a direct pathway to calculating the altitude. By rearranging the formula for the area of a triangle, which incorporates the base and the height (altitude), individuals can systematically solve for the altitude, thereby gaining a comprehensive understanding of the spatial dimensions within the triangle.

The area of a triangle can be calculated using various methods, with one of the fundamental formulas being A = 0.5 * base * height, where the base represents the length of the side to which the altitude is drawn. By rearranging this formula to solve for the height (altitude), individuals can obtain a precise measurement of the altitude. This method offers a straightforward and practical approach to finding the altitude of a triangle, providing a direct link between the area, base, and height of the triangle.

When the base and the corresponding height are known, individuals can apply the area formula to calculate the altitude, thereby gaining insights into the spatial relationships within the triangle. This method not only offers a systematic approach to determining the altitude but also enhances the understanding of the fundamental principles governing the spatial dimensions of geometric shapes. By leveraging the area formula, individuals can unlock the mysteries of altitude in triangles and apply this knowledge to practical scenarios in diverse fields.

In essence, using the area of the triangle to find the altitude offers a structured and insightful approach to understanding the perpendicular distance within a triangle. By rearranging the area formula to solve for the altitude, individuals can gain valuable insights into the spatial relationships and dimensions within the triangle, thereby enhancing their ability to solve geometric problems with precision and confidence. This method not only enriches the understanding of geometry but also equips individuals with practical tools for solving real-world problems that involve spatial configurations and distances.

Practical Applications of Finding the Altitude of a Triangle

The concept of finding the altitude of a triangle extends far beyond the realm of geometry, finding practical applications in diverse fields such as architecture, engineering, navigation, and surveying. By understanding the altitude of a triangle and its significance in spatial relationships, individuals can apply this knowledge to solve real-world problems and address practical challenges.

In architecture and engineering, the altitude of a triangle plays a crucial role in designing and constructing structures with precise measurements and dimensions. By determining the altitude of a triangular roof or the height of a triangular support structure, architects and engineers can ensure the structural integrity and stability of buildings. This application of altitude calculations enables professionals to create accurate blueprints and designs, leading to the construction of safe and reliable architectural marvels.

Moreover, in the field of navigation, the concept of altitude in triangles is utilized in celestial navigation and determining the height of objects. Navigators and pilots rely on altitude calculations to measure the angle between the horizon and a celestial body, such as the sun or stars, to determine their position and course. This application of altitude in navigation is essential for safe and precise travel, especially in maritime and aviation contexts.

Furthermore, the practical applications of finding the altitude of a triangle extend to surveying and land measurement. Surveyors utilize altitude calculations to determine the elevation of land, map out terrain, and establish boundaries. By employing the principles of altitude in triangles, surveyors can accurately measure the heights of natural features, such as mountains and cliffs, as well as man-made structures, facilitating urban planning and land development.

Additionally, the concept of altitude in triangles finds application in fields such as telecommunications and urban planning, where the determination of heights and distances is essential for optimizing signal transmission and designing efficient infrastructure.

In essence, the practical applications of finding the altitude of a triangle permeate various disciplines, offering valuable tools for solving real-world problems and addressing practical challenges. By leveraging the concept of altitude in triangles, professionals in architecture, engineering, navigation, surveying, and other fields can enhance their ability to make informed decisions, design precise structures, and navigate with accuracy, ultimately contributing to the advancement of diverse industries and the improvement of everyday life.

Conclusion

In conclusion, the concept of altitude in a triangle serves as a cornerstone in geometry, offering valuable insights into the spatial relationships and dimensions within triangles. By exploring the methods for finding the altitude of a triangle, including the application of trigonometry and the area formula, individuals can gain a comprehensive understanding of the perpendicular distance from a vertex to the opposite side. This knowledge not only enriches the understanding of geometric principles but also equips individuals with practical tools for solving real-world problems and addressing practical challenges in diverse fields.

The systematic application of trigonometry to find the altitude of a triangle provides a structured and precise approach to solving geometric problems. By leveraging the relationships between angles and sides, individuals can harness the power of trigonometric functions to determine the altitude, unlocking the spatial dimensions and relationships within triangles. This method not only enhances the understanding of the geometric properties of triangles but also equips individuals with the tools to solve real-world problems that involve spatial configurations and distances.

Furthermore, utilizing the area of a triangle to find the altitude offers a practical and insightful method for determining the perpendicular distance from a vertex to the opposite side. By rearranging the area formula to solve for the altitude, individuals can obtain a precise measurement of the altitude, thereby gaining a comprehensive understanding of the spatial dimensions within the triangle. This method not only offers a systematic approach to determining the altitude but also enhances the understanding of the fundamental principles governing the spatial dimensions of geometric shapes.

The practical applications of finding the altitude of a triangle extend to diverse fields such as architecture, engineering, navigation, and surveying. By understanding the altitude of a triangle and its significance in spatial relationships, individuals can apply this knowledge to solve real-world problems and address practical challenges. This application of altitude calculations enables professionals to create accurate blueprints and designs, leading to the construction of safe and reliable architectural marvels.

In essence, the concept of altitude in triangles permeates various disciplines, offering valuable tools for solving real-world problems and addressing practical challenges. By leveraging the concept of altitude in triangles, professionals in architecture, engineering, navigation, surveying, and other fields can enhance their ability to make informed decisions, design precise structures, and navigate with accuracy, ultimately contributing to the advancement of diverse industries and the improvement of everyday life.